Physics to Go!
Part 1
Interactive Quantum Mechanics
These are the basic controls of the app:
home page -
set the range of the x-value for which the Schrödinger equation is calculated (box size) with xmin, xmax
set basic step size for the time evolution
set the number of points of the wave function to be calculated. A larger number slows down the calculation and uses up more memory, but can increase the accuracy of the calculation.
pick from a list of predefined potentials or choose "user defined" and type a formula into the potential text field like, e.g., x*x .
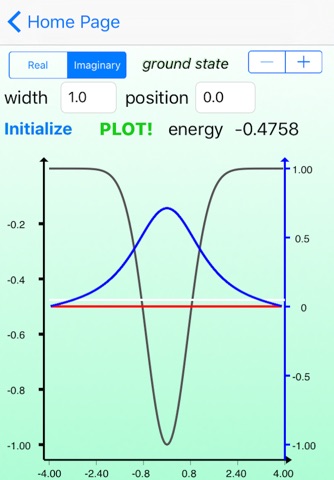
Pressing "Graph" brings you to the plotting window. The graph displays the potential, the wave function (blue real part, red imaginary part), and the energy of the system (indicated as white bar). The left y axis denotes the values of the potential and energy, the right one corresponds to the value of the wave function. "PLOT!” starts the plotting.
With the +/- stepper you can choose to calculate the ground state or one of the first nine excited states. Note that in order to get the correct, e.g., third excited state, you first have to calculate the ground, first and second excited state in this sequence.
You can choose between real time solution of the Schrödinger equation or imaginary time, which relaxes the state to the ground, or chosen excited, state. As you might observe, in a real time calculation, after a while the wave function tends to relax into these states as well. This is essentially due to an artefact of the numerical procedure for solving the equations, effectively introducing a small imaginary part to the time. In principle, with more effort this could/should be done better.
A few remarks about the underlying physics. The app solves the time-dependent Schrödinger equation, working best for imaginary time. Essentially, in the latter case it solves for the eigenstates and the corresponding eigenvalues of the energy of the system. For more on the math, interpretation, … there are infinitely many documents on the web, just look at the entry for the Schrödinger equation in Wikipedia or any other sites.
Try this with the app! You’ll observe some deviations, which is due to the imperfect numerical scheme and sometimes also because the calculation is done in a finite box in the x direction, setting the wave function to zero at the edges of the box. In spite of the apps shortcomings, it should still be quite entertaining and also instructive to play around with the app. For instance, look at the gaussian potential. You see that the excited states have energies higher than the potential. Thus, without finite box, the particle could not be kept in the potential well anymore but would escape. If you construct a very narrow potential well, this could even happen with the ground state of the system. Then, no state can be localised by the potential (although a classical particle at minimum energy would just sit at the lowest point of the potential well). This is a striking example of the effect of the quantum zero point energy. Thus, if you squeeze some solid states, which usually would form a crystal, more and more, going to very high densities, the potential well might narrow so that the ground state energy is above the potential, transforming the substance back again to a liquid at very high densities due to the zero-point motion even if the temperature of the system is at zero degree Kelvin (this might happen with lattices of atomic nuclei in the outer regions of neutron stars, for example)!
You can also observe, how the wave function of the particle leaks into x-ranges, which a classical particle could not access, and might even connect two separate potential wells (as in the double-well example), which is a case of the famous quantum tunnelling through barriers that could not be traversed in classical physics.
Enjoy the app!
